Abstract
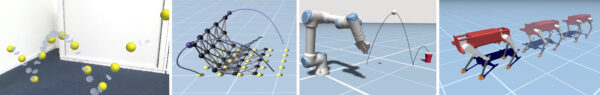
We present a differentiable dynamics solver that is able to handle frictional contact for rigid and deformable objects within a unified framework. Through a principled mollification of normal and tangential contact forces, our method circumvents the main difficulties inherent to the non-smooth nature of frictional contact. We combine this new contact model with fullyimplicit time integration to obtain a robust and efficient dynamics solver that is analytically differentiable. In conjunction with adjoint sensitivity analysis, our formulation enables gradient-based optimization with adaptive trade-offs between simulation accuracy and smoothness of objective function landscapes. We thoroughly analyse our approach on a set of simulation examples involving rigid bodies, visco-elastic materials, and coupled multibody systems. We furthermore showcase applications of our differentiable simulator to parameter estimation for deformable objects, motion planning for robotic manipulation, trajectory optimization for compliant walking robots, as well as efficient self-supervised learning of control policies.
Additional Content
Copyright Notice
The documents contained in these directories are included by the contributing authors as a means to ensure timely dissemination of scholarly and technical work on a non-commercial basis. Copyright and all rights therein are maintained by the authors or by other copyright holders, notwithstanding that they have offered their works here electronically. It is understood that all persons copying this information will adhere to the terms and constraints invoked by each author’s copyright. These works may not be reposted without the explicit permission of the copyright holder.